Pythagoras' law of harmony

Once upon a time, there were four little factoids rolling around in John's brain. Being in such a tiny and empty space, they were bound to eventually bump into each other. And when they did, it was cause for celebration, what with the whole being greater than the sum of the parts and all that.

Factoid #1, Pythagoras' law of harmony

Pythagoras stated a simple law to explain why certain notes sound harmonious: the ratios of their frequencies are in simple integer ratio. He didn't quite put it in those words, but that's the modern description. Two notes that are a major third apart sound good because the ratio of their frequencies are 5 to 4. For those who don't remember, I expounded on this law of harmony in a previous blog post about tuning pianos.

This has always been a little confusing for me. Not much, but a little. Why is the ear so incredibly sensitive to these ratios?  If you play a note, any decent singer can sing a note twice or three or four times as high to within a few per cent. Can you judge weight that well? How accurately can you estimate three inches after being shown one inch?

Factoid #2, Tuned cilia in the cochlea

We sense notes because of little hairs in our ears. I am not talking about those unkempt hairs that totally gross my wife out... I mean little, itty-bitty hairs that are deep in the ear and well beyond where any self-respecting Q-tip should ever go, inside the cochlea. 

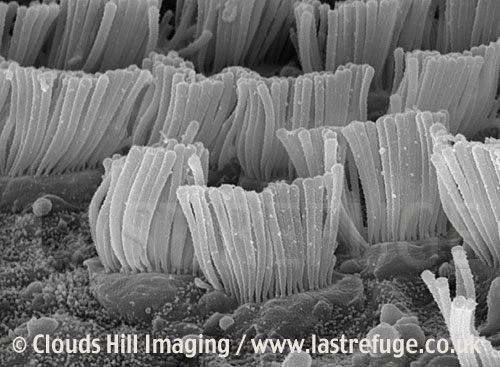
Way cool SEM image of cilia from Clouds Hill Imaging www.lastrefuge.co.uk

Those cilia dance to the music, but they wiggle around only when they happen on a frequency that they are tuned to. Each cilia is connected to a nerve, and what we sense as a tone is the reaction to that cilia dance. For those who don't remember, I expounded on the inner working of the ear in a blog post about being tone deaf.

Based on this, it again seems odd to me that we should be so darn precise in our assessment of an octave. Are the cilia really tuned that precisely?

Factoid #3 - Tartini tones

It is painful to admit, but at one time I did sing in a barbershop chorus. In fact, I actually organized a quartet while in high school. That's me, sporting the Fu Manchu in the picture from our yearbook of the Thundertones. Our high school mascot was the Thunderbird, hence the name. But, the name was also a play on the word "undertone". Barbershoppers are always talking about overtones, which were notes that are heard which are way above anything that anyone is actually singing. Undertones are just the opposite. 

From right to left, Denny, Kenny, Johnnie and Chip, AKA The Thundertones

Roger was the director of a barbershop chorus that I was in. A chorus, by the way, is a whole bunch of guys singing barbershop together. Roger came to rehearsal one night all excited to play with undertones. He had the chorus sing a set of very specific notes, each part with a specific volume. The result was that a magic note appeared, a note which no one was singing. The note was somewhere deep and dark in the basement where only the bassest of the basses have ever visited. We're talking Johnnie Cash, Tennessee Ernie Ford, and Lurch.

This phenomena of creating low notes from higher ones was discovered by a violinist by the name of Giuseppe Tartini, so they go by the name of Tartini tones. They can be used in the design of pipe organs as well. A combination of a 16 foot pipe and a 10 foot 8 inch pipe can be made to sound like a 32 foot pipe, thus saving a lot of space and expense. These pipes are called helper ranks.

For those of you having trouble hearing them, you might want to try liberal application of libations. This will bring out the Martini tones.

I have seen this in audio processing as well. I recorded a tone, and edited the waveform to remove the fundamental frequency (using fast Fourier transforms). When I played back the filtered waveform, it sounded like the fundamental frequency was still there.

So... what gives with that? I thought the ear was designed to pull out frequencies?!?!? How does my ear get fooled?

Factoid #4 - Overtones and vibration modes

Sympathetic vibration and overtones can be used to explain the stupid piano tricks that I once blogged about. In the example below, middle C is silently held down so that the wire is free to vibrate. The F below this is plunked and then let up to silence this note. The note that is sustained is the C above middle C.

One of many stupid piano tricks that illustrate overtones and vibration modes

What's happening is that the F note generates a series of overtones. It likes to vibrate at F below middle C, but also at twice the frequency (F above middle C), and also at three times the frequency (C above middle C). 

Meanwhile, the middle C wire doesn't like to vibrate at F below middle C, nor at F above middle C. But, C above middle C is in it's overtones series. So, when the plunked note is let up, the middle C wire continues to sound the C above middle C. 

Here are the two basic rules to remember: 1. Things that vibrate easily at one frequency tend to like to vibrate easily at twice that frequency, and three times that frequency, and four times, etc. 2. In order for resonance to happen, the sender must send out wavelengths that the receiver likes.

A sudden insight

As I said, these four factoids happen to collide in my brain one day. I think Factoid #4 is the explanation of the conundrums that I had with the first three factoids.

Let's just say a middle C just happens to crawl into my cochlea. All by itself. Normally, it will come in with an entourage of overtones, but let's just say this tone was produced by lightly blowing on a soda bottle. Pure middle C.

Now, if you didn't think about it for too long, you would expect that only one set of cilia would vibrate. I mean, that's kinda the design goal, isn't it? Each cilia is supposed to be some kind of tuning fork sensor for middle C or the B flat two octaves below middle C, or whatever. Right?

But remember the first rule of overtones and vibration modes. A single frequency will likely get several sets of cilia going. Middle C will activate the middle C cilia, but the cilia tuned for an octave below will also set to vibrating. Clearly they won't vibrate really strongly, or we would always hear that lower octave. But, if you get enough of the overtones going, and you will hear that Tartini tone. That explains factoid #3.

Factoid #2 is explained by noting that two notes that are exactly an octave apart will stimulate the same sets of cilia. The brain doesn't have to try to map each cilia to a frequency and then calibrate each one accurately. The two notes sound very similar because they activate some of the same cilia.

How about factoid #1?  The answer is pretty much the same. Because simple ratios fit in well with the overtone series, and sets of cilia are inextricably linked through the overtone series, notes with simple ratios sound good together. They stimulate the same sets of cilia.

I don't know about you, but I'm feeling pretty good about this. I'm gonna treat myself to the Midwest Vocal Express concert. Best barbershop music in the Milwaukee area. The concert is Saturday, May 10, with shows at 2:00 PM and 7:30 PM, at the Nathan Hale Auditorium, 11601 W Lincoln Ave., West Allis, Wisconsin.